Fig.5 (left) shows that, after ablation, the fibers and matix emerge from the surface and have a characteristic shape with an approximate tip angle of 30°. The main cause of this morphology is the presence of a weak interphase between the fibers and the intra-bundle matrix, associated to a low Da number.
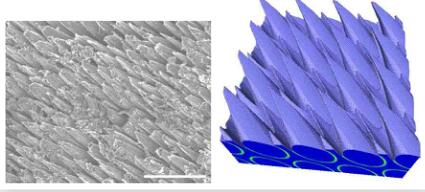
fig.5-left-SEM-micrograph-of-the-ablated-surface-of-a-bundle-in-4D-CC-composite.
In those conditions, it has been shown that the effetive reactivity of the bundle is close to the one of the weakest phase, that is, of the interphase. This has been taken as a first clue to the identification of the individual reactivities of all phases, together with the assumption that the matrix has approximately the same reactivity as the fibers. The ratio between fiber and interphase reactivity has been varied systematically and the resulting steady-state angle tip was measured; a correct match between experimental and modelled morphologies has been obtained for a ratio close to 8.5. Then, it has been confirmed that the effective reactivity of the bundle is very close to the reactivity of the weakest phase. It has also been checked that the transient time (≈3s) before development of the steady morphology is very short with respect to the total rocket firing time in usual tests.
Using these results, bundle-scale modelling is possible with our developed code by using the obtained effective reactivity. However, we know that turbullent effects at this scale might be important. To take into account these effects without having a strong modification of the mass transfer model, the diffusivity coefficient is artificially increased in order to represent the turbulent diffusivity. Values were matched to the turbulent kinematic viscosities obtained by a 1D boundary layer code. One missing input parameter is the reactivity ratio between the bundle and the inter-bundle matrix phases. Again, a simulation is run several times with a variation of the reactivity contrast, until the correct morphology is reproduced. The resulting evolution of the composite is represented in Fig.6. One observes that, after a strong change in the composite surface, the roughness amplitude reaches a steady state. To obtain the very satisfactory agreement shown in Fig.7. the reactivity ratio between bundles and matrix is found to be 1/2. This means that the weakest phase at the macroscopic scale is twice as reactive as the strongest one.

fig.7-comparison between simulated (left) and experimental (right) structures.
An agrument for this surprising agreement is the following. The total resistance to ablation is the result of several phenomena chained together in series: first, the heterogeneous reaction; second, the diffusion through the mass transfer boundary layer, which can be equated to the laminar bounday layer; third, mass transfer by convection and diffusion through the turbulent fluid. The most important resistances control the ablation rate as a whole. It appears that the transfer resistance in the turbulent fluid is not important. The controlling phenomena are then the heterogeneous reaction and the transfer through the laminar boundary layer. For the latter, the only critical parameter is the boundary layer thickness, that can be extracted from specific computations. So, reducing the model to heterogeneous reaction and pure diffusion through a laminar boundary layer still allows to capture the main features of the wall recession dynamics.
Composites with woven architecture:
Another class of C/C composites is prepared from preforms made of woven carbon fiber fabrics. The matrix is infiltrated between the fibers by Chemical Vapor Infiltration, i.e., a gas-phase route. During this process, the tortuosity of the preform plays an important role by limiting the deposition in the composite core. As a consequence, the composite material at the end of the process still contains about 10% pore volume. In this section, DiAbl3D is used to investigate the role of the tortuosity of two different composites on their effective ablative behavior. Since the developed model cannot describe precisely the turbulent transfer occuring in the nozzle, the ablation process modeled here refers more to a purely diffusive oxidation of the material, e.g. in a laboratory furnace, than to the nozzle application. This study has been made for different Damkohler numbers. For a given composite, this dimensionless number is a function of temperature because of the exponential variation of the reactivity. The Damkohler values used in the simulation are therefore linked to the temperature of the experimental furnace.